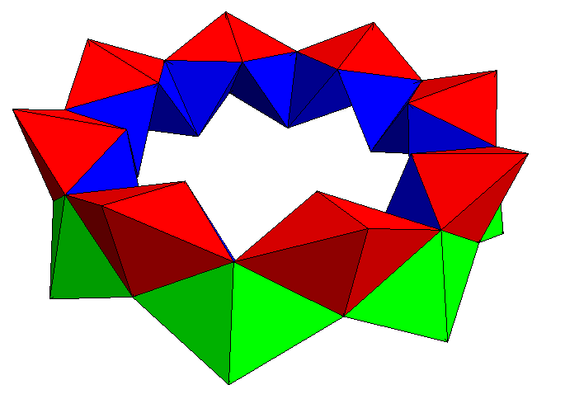
Ping-coincidence lattice "with a twist":
a "Null-Strut Wheel".
"Can this set of causal relations (with 8-fold rotational spatial symmetry) be found in a spacetime region of Schwarzschild geometry ?"
(PSE/q/845793)
(Enjoy it while it out-lasts the drive-by-assaulting ...)
#PingCoincidenceLattice #NullStrutWheel #Spacetime #Relativity
@lindsey
Tangent2 (incl. note to myself):
I've been thinking/writing/advocating #PingCoincidence #PingCoincidenceLattice #PingDuration (cmp. in German "Ping-Koinzidenz" "Ping-Koinzidenz-Gitter" "Pingdauer") as basic construction or method of measurement in #Relativity implementing [Einstein's maxime](http://einsteinpapers.press.princeton.edu/vol6-trans/165?highlightText=coincidences)
[Your article](https://decomposition.al/blog/2023/01/18/enforcing-causally-ordered-message-delivery-on-the-senders-side/) uses the term "ack" ("#acknowledgement"); correspondingly #AckCoincidence #AckCoincidenceLattice
but not "AckDuration" !
(1/2)
@ocfnash
http://olivernash.org/2018/07/08/poring-over-poncelet/index.html
Awesome!
I'd love to find out about #Poncelet generalizations or related results in 3+1 dimensional flat #MinkowskiSpace, with
- all relevant edges along light cones (Are those "singular" and perhaps problematic, even in 3+1 D ?), and
- the \(n\)-sided polygon generalized to a #PingCoincidenceLattice (cmp. my sketch https://mathstodon.xyz/@MisterRelativity/109435130217990848 )
Neat!
Related question:
Attaching \(n\) equal tetrahedra face-to-face in some 3D-sequence, how close (in terms of a positive fraction of edge length) can two vertices be, as a function of \(n\) ?
