This uses rigid rods. Seems obvious in hindsight?
#MathArt #geometry #animation #loop #geogebra #octahedron #cube #3d

This is what I was trying to do originally, I wonder what it would look like if I used rigid rods for the edges.
#MathArt #geometry #animation #loop #geogebra #octahedron #cube #3d

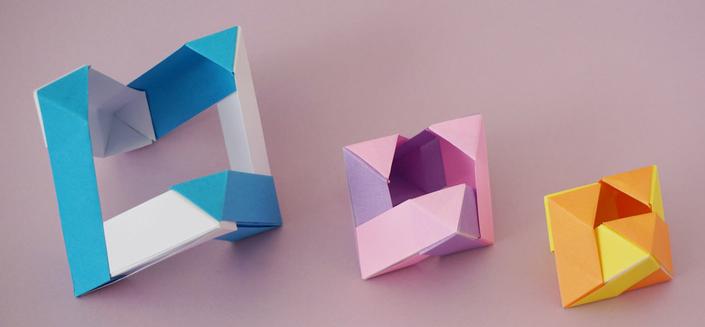
Three modular origami octahedra made from three different kinds of rectangles (Canoe Unit 60° by me).
From left to right, the rectangles are 1:√2, square and 2:√3.

#origami @origami #MathArt #geometry #octahedron #ModularOrigami #papercraft #craft

@mrdk @unnick This version shows how the cube/octahedron works using a rhombic dodecahedron (without scaling the bars to have constant length).
#MathArt #geometry #animation #loop #geogebra #cube #octahedron #3d

@mrdk @unnick
I'm not sure that these are related to the Jitterbug transformation.

This is my recreation of unnick's original cube/octahedron loop. I used the rhombic dodecahedron and rhombic triacontahedron for this and the previous loop. They remind me of tensegrity structures.

BTW, I made a couple of origami versions of the Jitterbug transformation many years ago. This one https://foldworks.net/wp-content/uploads/2018/06/jitterbug.pdf works better than the first version https://britishorigami.org/academic/davidpetty/origamiemporium/lam_jitterbug.htm

#MathArt #geometry #animation #loop #geogebra #cube #octahedron #3d #Jitterbug #origami

Very special and rare Blue #Octahedron #Spinel with natural growth marks from #SriLanka.
Photo: Ben Decamp Bill Larson Collection
By #GeologyWonders
#Geology #GeologyPage #Nature #Minerals #Crystals #Gems #Nature #NatureLovers #NaturePhotography #Photographer #RockPorn

I took a look at the dual polytope to \(\omega^{xx,e}_{6}\) - i.e., \(\omega^{xx,e*}_{6}\). The dual does help to understand the polytope's structure, I think.
To get the dual polytope, I derived the polytope having facet-vertex incidence matrix = the transpose of the facet-vertex incidence matrix of \(\omega^{xx,e}_{6}\). So, its vertices correspond to the facets (and facets to the vertices of) \(\omega^{xx,e}_{6}\).
The picture below is not the flux capacitor from BTTF, but a diagram of \(\omega^{xx,e*}_{6}\) that I call a tetra-oct. The diagram is a tetrahedron, but instead of edges, there are octahedra. (I made the octahedra oblong & used a Schlegel perspective to make it easier to draw.) This diagram shows all 28 vertices, 72 of the 240 edges, and all 6 of the octahedron faces of \(\omega^{xx,e*}_{6}\).
\(\omega^{xx,e*}_{6}\) is 9-dimensional, it has four 6-d tri-oct faces, and six (3-d) octahedron faces! The octahedra are regular-ish, with parallel antipodal edges.
The four (0-d) corners of the tetrahedron correspond to the four \(\omega^{xx,e}_{6}\) (8-d) facets which are three parallelograms in general position. The six (3-d) octahedra correspond to the six \(\omega^{xx,e}_{6}\) (5-d) faces which are two parallelograms in general position. The four 6-d tri-oct faces correspond to the four 2-d parallelogram faces of \(\omega^{xx,e}_{6}\). It appears \(\omega^{xx,e*}_{6}\) is equifacetted (all its facets have the same combinatorial type); intuitively appealing as the 16 facets correspond to the even permutations among the vertices of \(\Omega^{xx}_{6}\).
#octahedron

"Rotations"

Tooll3 is really fantastic as soon as you start to write your own operators.
It is so easy and opens so many doors.
Here I use my chromatic aberration shader with continuously changing displacement angles in a feedback loop for amazing, prism like colours.

https://youtu.be/iCo3UCNQWHQ

#Tooll3 #realtime #newmediaart #abstractart #generativeart #particles #livingpainting #creativecoding #hlsl #gpu #shader #generative #procedural #visualart #chromaticaberration #mastoart #octahedron #flow