Change my mind: pseudogroups are the "wrong way" to formalize differential geometry.
What's wrong with formalizing charts and atlases, then a manifold is a set equipped with a maximal atlas?
We could formalize, e.g., complex manifolds using G-structures.
What is wrong with this approach?
Differential Geometry: A First Course in Curves and Surfaces [pdf]
https://math.franklin.uga.edu/sites/default/files/users/user317/ShifrinDiffGeo.pdf
#HackerNews #DifferentialGeometry #Curves #Surfaces #MathEducation #PDF #Resources
Got a reprint of this classic earlier this month. Good memories of learning exterior products for the first time from this. I wish/hope someone writes a biography of Spivak, always fascinates and inspires to know about people who dedicated their life to see the universe through the keyhole of a specific area. Spivak did that for all things related to geometry. A rare persona, and much rarer today.
Real-life application of the Theorema Egregium https://en.wikipedia.org/wiki/Theorema_Egregium
'time is a flat circle'? what are you talking about, all circles are flat
Equivalent latitude (Climatology 🌍)
In differential geometry, the equivalent latitude is a Lagrangian coordinate. It is often used in atmospheric science, particularly in the study of stratospheric dynamics. Each isoline in a map of equivalent latitude follows the flow velocity and encloses the same area as the latitude line of equivalent value, hence "equivalent latitude."...
https://en.wikipedia.org/wiki/Equivalent_latitude
#EquivalentLatitude #Climatology #DifferentialGeometry #Equivalence
(2/4)
… And for a normal (i.e. \( \mathbb R \)-valued) diff form the cov ext diff \( d_\nabla \) shall be just the same as the normal ext diff \( d \).
This confuses me for a long time.
Until I realised: the eq I wrote above was taken from a #GR textbook, and physicists tend to write every things into coordinates/components/infices format, which brings the confusion.
#DifferentialGeometry #Gravity #GeneralRelativity #Mathematics #Physics
(3/4)
In fact, the tetrad shall be considered as a vector valued 1-form:
\[
theta = \theta_j^i \partial_i \otimes d x^j,
\]
therefore there is no meaning for the cov ext diff for a component of a vector!
One should really consider is the \( d_\nabla \theta\), where the connection is considered on the tangent bundle of the spacetime manifold.
#DifferentialGeometry #Gravity #GeneralRelativity #Mathematics #Physics
(4/4)
I just really hate how physicist write the cov derivative of the *components* of a tensor (or a section of any vector bundle), while it only make sense if you consider the derivative of the tensor itself.
#DifferentialGeometry #Gravity #GeneralRelativity #Mathematics #Physics
(1/4)
I was trying to reason about the (1st) Cartan structure equation
\[ d_\nabla \theta^i = d \theta^i + \Gamma^i_j \wedge \theta^j = 0,\]
where \( d_\nabla \) is the covariant exterial differential, \(\nabla\) is the Levi-Civita connection with connection form \( \Gamma \), and \( \theta \) is an (orthogonal) tetrad.
For me this does not make sense, since \( \theta^i \) is just a normal 1-form, and…
#DifferentialGeometry #Gravity #GeneralRelativity #Mathematics #Physics
Whenever I walk to/from home, I have to walk up/down an inclined street; I noticed that the asphalt floor has different curvatures depending on how near it is of a bend, and I try to find a less steep incline while walking.
This got me inspiration for the few questions below. Any simple explanations, and related links, are welcome.
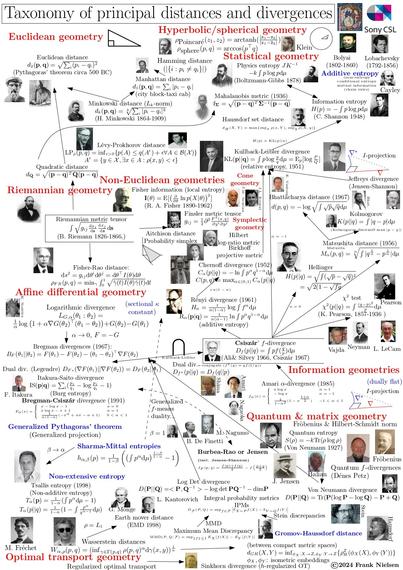
Given a #differentiable surface within R^3, and two distinct points in it, there are infinitely many differentiable paths from one point to another, remaining on the surface. At each point of the #path, one can find the path's local #curvature. Then:
- Find a path that minimizes the supreme of the curvature. In other words, find the "flattest" path.
- Find a path that minimizes the variation of the curvature. In other words, find a path that "most resembles" a circle arc.
Are these tasks always possible within the given conditions? Are any stronger conditions needed? Are there cases with an #analytic solution, or are they possible only with numerical approximations?
#Analysis #DifferentialGeometry #Calculus #DifferentialEquations #NumericalMethods
Taxonomy of principal distances and divergences!
Poster on the taxonomy of main statistical distances with their underlying geometries! Credit:
Frank Nielsen; PDF 🔗 https://franknielsen.github.io/GSI/Poster-Distances.pdf
#EuclideanGeometry #HyperbolicGeometry #SphericalGeometry #StatisticalGeometry #RiemannianGeometry #NonEuclideanGeometry #DifferentialGeometry #AffineDifferentialGeometry #InformationGeometry #QuantumGeometry #MatrixGeometry #Taxonomy #PrincipalDistance #Divergence #Distance #Generalization
Ordered this book for a Mathematician at work. Can anyone explain how this relates to 'differential geometry'?!??? Possibly the most baffling book cover I have ever seen. Can anyone offer any sort of explanation? Or is it just that thing where STEM textbooks have nonsensical covers because images can't really sum up what the book is about? I am so intrigued
#bookCovers #STEM #geometry #differentialGeometry #midcentury #maths #math #mathematics
From "Fundamentals of Differential Geometry" by S. Lang (1999)
https://link.springer.com/book/10.1007/978-1-4612-0541-8?utm_source=dlvr.it&utm_medium=mastodon
Have you seen the latest article from our workshop (non-regular #spacetime #geometry) participants? 👉
Saúl Burgos, José Luis Flores and Jónatan Herrera: The c-completion of Lorentzian metric spaces
#SpacetimeGeometry
#DifferentialGeometry #GeneralRelativity #QuantumCosmology #MathematicalPhysics #Quantum #Cosmology #Relativity #MetricGeometry
@univienna
https://arxiv.org/pdf/2305.02004.pdf
(for the motion picture please visit https://twitter.com/ESIVienna/status/1671167715313344512)
Have you seen the latest article by Brian Allen, a former workshop participant?
#Spacetime #Geometry #SpacetimeGeometry
#DifferentialGeometry #GeneralRelativity #QuantumCosmology #MathematicalPhysics #Quantum #Cosmology #Relativity #MetricGeometry
@univienna
Check out the newest results on #Finsler #Spacetimes by our visitors! 🧐
#DifferentialGeometry #GeneralRelativity #QuantumCosmology #MetricGeometry
I've worked out that the injectivity radius under the Euclidean metric for the #unitary group U(n) is π and for real and special subgroups O(n), SO(n), and SU(n) is π√2.
This seems like a pretty basic property, but I can't find a single reference that gives the injectivity radii for any of these groups. Anyone know of one?
"Riemann’s Seminal Lecture on Non-Euclidean Geometry" by Areeba Merriam 👉 🔗 https://www.cantorsparadise.com/reimanns-seminal-lecture-on-non-euclidean-geometry-11673a8d6fbb
#Riemann #SeminalLecture #NonEuclideanGeometry #EuclideanGeometry #Gravity #DifferentialGeometry #Relativity #GeneralRelativity #SpecialRelativity #RiemannianGeometry #Tensor #Minkowski #HermannMinkowski #Spacetime #Space #Time #Curvature #Klein #Jacobi #Dirichlet #Gauss #Hypersurface #EllipticGeometry #Geometry #Physics #Topology #Hyperspace #Einstein #Isaacson #Kaku #CantorsParadise
