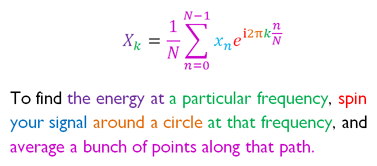So next time you're running a Fourier transform or analyzing frequency components, give a little nod to Schuster. 👏
#DataScience #SignalProcessing #TimeSeries #SpectralAnalysis #FourierTransform #TechHistory #Analytics
---
#FourierTransform
“xQc couldn’t believe Fourier Transformation” — “xQc non riesce a credere alla Trasformata di Fourier”
https://www.youtube.com/watch?v=oL1gHyuPOsANel corso di reti all’università è stata menzionata la trasformata di #Fourier… per fortuna solo menzionata, perché giustamente il corso da noi si fa pur sempre solo dal punto di vista informatico, non ingegneristico… e boh, ricordo che ho dormito per tutto il misero minuto per cui la diapositiva a riguardo è rimasta a schermo. Non voglio assolutamente avere a che fare con queste formule complicate strane, altrimenti sarei andata ad ingegneria informatica come altri miei amici…
…Però, devo dire che guardando l’argomento con l’animazione bellina, la formula messa effettivamente in un contesto dimostrativo ben illustrato, e quindi insomma questo tipo di vibe qui, il mio rifiuto si fa meno forte. Personalmente a guardare questa clip comunque non ho reagito come invece si vede #xQc fare qui; grande streamer lui, che non immaginavo avesse sviluppato questa particolare passione per la #matematica… anche se lui è talmente sbigottito alla vista della trasformata di Fourier che addirittura non ci ha potuto nemmeno credere. DAMN indeed, that is in fact insane, caro mio…
The Fourier Transform is a mathematical operation that transforms a function of time (or space) into a function of frequency. It decomposes a complex signal into its constituent sinusoidal components, each with a specific frequency, amplitude, and phase. This is particularly useful in many fields, such as signal processing, physics, and engineering, because it allows for analysing the frequency characteristics of signals. The Fourier Transform provides a bridge between the time and frequency domains, enabling the analysis and manipulation of signals in more intuitive and computationally efficient ways. The result of applying a Fourier Transform is often represented as a spectrum, showing how much of each frequency is present in the original signal.
\[\Large\boxed{\boxed{\widehat{f}(\xi) = \int_{-\infty}^{\infty} f(x)\ e^{-i 2\pi \xi x}\,\mathrm dx, \quad \forall\xi \in \mathbb{R}.}}\]
Inverse Fourier Transform:
\[\Large\boxed{\boxed{ f(x) = \int_{-\infty}^{\infty} \widehat f(\xi)\ e^{i 2 \pi \xi x}\,\mathrm d\xi,\quad \forall x \in \mathbb R.}}\]
The equation allows us to listen to mp3s today. Digital Music Couldn’t Exist Without the Fourier Transform: http://bit.ly/22kbNfi
#Fourier #FourierTransform #Transform #Time #Frequency #Space #TimeDomain #FrequencyDomain #Wavenumber #WavenumberDomain #Function #Math #Maths #JosephFourier #Signal #Signals #FT #IFT #DFT #FFT #Physics #SignalProcessing #Engineering #Analysis #Computing #Computation #Operation #ComplexSignal #Sinusoidal #Amplitude #Phase #Spectra #Spectrum #Pustam #Raut #PustamRaut #EGR #Mathstodon #Mastodon #GeoFlow #SpectralMethod
And then we have folks that said "I'll never use math in life again". Right 😁
💡 Did you know that #FluidFFT lets you do much more than computing #FourierTransform and its inverse?
With an "OperatorsPseudoSpectral2D" (or 3D) class you can compute transforms, compute derivatives, divergence, curl, gradients, apply dealiasing etc easily and efficiently!
You don't have to grok how FFTs are arranged numerically and what wave numbers are. It simplifies things. Here is an example from the archives
https://fluiddyn.netlify.app/intensely-edgy-cat-with-fluidfft
https://fluidfft.readthedocs.io/en/latest/generated/fluidfft.fft2d.operators.html
Since "jct." is short for "junction," every time I read "DCT" (discrete cosine transform) I hear "dunction."
#DSP #Audio #DigitalAudio #Math #FourierTransform #Maps #Mapstodon
An Interactive Guide to Fourier Series.
#genuary31 - "Generative Audio"
The final day of Genuary is "Generative Audio". Instead of trying to generate an audio clip, I used an audio clip to generate an image. Many different clips within a recording of Beethoven's Pathétique Sonata were passed through an FFT with smoothing and coloring applied.
I've archived all of my Genuary posts on my website: https://codeismycanvas.art/posts/genuary24/
#genuary #beethoven #audiovisualizer #fft #fouriertransform #generativeart
How Shazam recognizes songs so quickly.
https://www.cameronmacleod.com/blog/how-does-shazam-work
#programming #computerscience #digitalaudio #fouriertransform
Mee-owch!
Groundbreaking Quantum Leap: Physicists Turn Schrödinger’s Cat on Its Head
Researchers have developed a groundbreaking method to perform the fractional Fourier Transform of optical pulses using quantum memory. This unique achievement involved implementing the transformation on a “Schrödinger’s cat” state, having potential applications in telecommunications and spectroscopy.
https://scitechdaily.com/groundbreaking-quantum-leap-physicists-turn-schrodingers-cat-on-its-head/ #fractional #FourierTransform #optical #pulses #quantum #memory #Schrödinger’sCat
The FFT nicely confirms the aperiodicity and an interesting 12-fold symmetry #Fouriertransform #crystallography
Its #Fouriertransform nicely shows the single handedness of the 4-fold axis; what I honestly did not expect are all those sharp peaks!
The Fourier transform (FT), explained in one sentence: 🔗 https://blog.revolutionanalytics.com/2014/01/the-fourier-transform-explained-in-one-sentence.html
\[\boxed{\hat{f}(\xi)=\displaystyle\int_{-\infty}^\infty e^{-i2\pi\xi t}f(t)\ \mathrm{d}t}\]
Discrete Fourier transform (DFT):
\[\displaystyle x_n = \sum_{k=0}^{N-1} X_k \cdot e^{-i2\pi \tfrac{n}{N}k}\]
Inverse transform:
\[\displaystyle X_k = \frac{1}{N} \sum_{n=0}^{N-1} x_n \cdot e^{i2\pi \tfrac{n}{N} k}\]
#FourierTransform #FourierSeries #Transform #MathematicalTransform #Signal #SignalProcessing #Frequency #Energy #FourierAnalysis #Series #Analysis
"Improving Spectral, Spatial, and Mechanistic Resolution Using Fourier Transform Nonlinear Optics: A Tutorial Review"
ACS Phys. Chem Au 2022
https://doi.org/10.1021/acsphyschemau.2c00051
#openaccess #Fouriertransform #Kineticparameters #Lasers #Nonlinearoptics #Plasmons
…time to get inspiration for some other #FourierOptics #Python tutorials to follow on from this, which remains the most popular post on the blog, by far
My favourite textbook of all time – had been in storage for a while as we did a long, convoluted, house move…
It’s back, safe and sound, nicely weathered and thumbed from all those years of use…
PS: just realised it’s my wife’s copy, not mine. I Want My One Back! (It’s still in a box, somewhere…)
#Python #Fourier #FourierTransform #FourierOptics #programming
Client Info
Server: https://mastodon.social
Version: 2025.04
Repository: https://github.com/cyevgeniy/lmst